Notice
Recent Posts
Recent Comments
Link
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | ||
| 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| 13 | 14 | 15 | 16 | 17 | 18 | 19 |
| 20 | 21 | 22 | 23 | 24 | 25 | 26 |
| 27 | 28 | 29 | 30 |
Tags
- 릿코드
- leetcode 풀기
- 알고리즘풀이
- 파이썬알고리즘
- 릿코드 풀기
- 잇츠디모
- 릿코드풀기
- 알고리즘풀기
- 파이썬릿코드풀기
- python 릿코드
- python priority queue
- python Leetcode
- python zip_longest
- 상가수익률계산기
- 코틀린기초
- binary search
- python 알고리즘
- 파이썬릿코드
- 릿코드풀이
- 릿코드 파이썬
- 파이썬 알고리즘 풀기
- LeetCode
- python xor
- leetcode풀기
- python sorted
- 파이썬 릿코드
- leetcode풀이
- 파이썬 알고리즘
- 파이썬 프로그래머스
- 파이썬알고리즘풀기
Archives
- Today
- Total
소프트웨어에 대한 모든 것
1834. Single-Threaded CPU 본문
반응형
제목
문제)
You are given n tasks labeled from 0 to n - 1 represented by a 2D integer array tasks, where tasks[i] = [enqueueTimei, processingTimei] means that the ith task will be available to process at enqueueTimei and will take processingTimei to finish processing.
You have a single-threaded CPU that can process at most one task at a time and will act in the following way:
- If the CPU is idle and there are no available tasks to process, the CPU remains idle.
- If the CPU is idle and there are available tasks, the CPU will choose the one with the shortest processing time. If multiple tasks have the same shortest processing time, it will choose the task with the smallest index.
- Once a task is started, the CPU will process the entire task without stopping.
- The CPU can finish a task then start a new one instantly.
Return the order in which the CPU will process the tasks.
Example 1:
Input: tasks = [[1,2],[2,4],[3,2],[4,1]]
Output: [0,2,3,1]
Explanation: The events go as follows:
- At time = 1, task 0 is available to process. Available tasks = {0}.
- Also at time = 1, the idle CPU starts processing task 0. Available tasks = {}.
- At time = 2, task 1 is available to process. Available tasks = {1}.
- At time = 3, task 2 is available to process. Available tasks = {1, 2}.
- Also at time = 3, the CPU finishes task 0 and starts processing task 2 as it is the shortest. Available tasks = {1}.
- At time = 4, task 3 is available to process. Available tasks = {1, 3}.
- At time = 5, the CPU finishes task 2 and starts processing task 3 as it is the shortest. Available tasks = {1}.
- At time = 6, the CPU finishes task 3 and starts processing task 1. Available tasks = {}.
- At time = 10, the CPU finishes task 1 and becomes idle.
Example 2:
Input: tasks = [[7,10],[7,12],[7,5],[7,4],[7,2]]
Output: [4,3,2,0,1]
Explanation: The events go as follows:
- At time = 7, all the tasks become available. Available tasks = {0,1,2,3,4}.
- Also at time = 7, the idle CPU starts processing task 4. Available tasks = {0,1,2,3}.
- At time = 9, the CPU finishes task 4 and starts processing task 3. Available tasks = {0,1,2}.
- At time = 13, the CPU finishes task 3 and starts processing task 2. Available tasks = {0,1}.
- At time = 18, the CPU finishes task 2 and starts processing task 0. Available tasks = {1}.
- At time = 28, the CPU finishes task 0 and starts processing task 1. Available tasks = {}.
- At time = 40, the CPU finishes task 1 and becomes idle.
Constraints:
- tasks.length == n
- 1 <= n <= 105
- 1 <= enqueueTimei, processingTimei <= 109
솔루션1)
- TLE 발생
class Solution:
def getOrder(self, tasks: List[List[int]]) -> List[int]:
# tasks with idx
tasks = [[idx] + task for idx, task in enumerate(tasks)]
tasks = sorted(tasks, key=lambda x: (x[1], x[2]))
# initial current time
cur_time = tasks[0][1]
ret = []
while len(tasks) > 0:
candidates = [[idx] + task for idx, task in enumerate(tasks) if task[1] <= cur_time]
# print(f'candidates : {candidates}')
if candidates:
# by processing time, by index
candidates = sorted(candidates, key=lambda x: (x[3], x[1]))
# print(f'sorted candidates : {candidates}')
cur_time += candidates[0][3]
ret.append(candidates[0][1])
# print(f'ret : {ret}')
del tasks[candidates[0][0]]
else:
cur_time = tasks[0][0]
return ret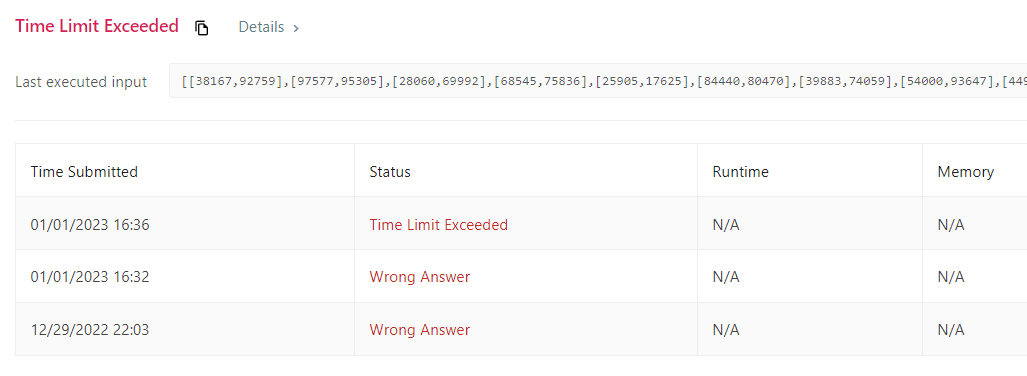
솔루션2)
- 솔루션1에서 처리해야 할 태스크를 매번 정렬하는 부분이 오버헤드로 판단
- heapq를 사용해서 추가되는 태스크만 정렬하도록 코드 최적화. heapq 정렬시 키는 processingTime, taskIdx를 사용해서 정렬
- TLE는 발생하지 않고 Success는 했지만 여전히 하위 5.72%f로 개선 여지가 많음
class Solution:
def getOrder(self, tasks: List[List[int]]) -> List[int]:
EUQUEUE_TIME_IDX = 0
PROCESSING_TIME_IDX = 1
TASK_IDX = 2
# tasks with idx
tasks = [task + [idx] for idx, task in enumerate(tasks)]
# enqueueTime 오름차순 정렬
tasks = sorted(tasks, key=lambda x: x[EUQUEUE_TIME_IDX])
# 초기 시간 설정
cur_time = tasks[0][EUQUEUE_TIME_IDX]
ret = []
# [key, task]
candidates = []
while len(tasks):
# 시간이 지나서 처리해야 할 태스크 후보 등록
while tasks and tasks[0][EUQUEUE_TIME_IDX] <= cur_time:
task = tasks[0]
key = f'{str(task[PROCESSING_TIME_IDX]).zfill(10)}_{str(task[TASK_IDX]).zfill(6)}'
heapq.heappush(candidates, [key, task])
# print(f'heap push - key : {key}, task : {task}')
del tasks[0]
# 태스트 처리
if candidates and candidates[0][1][EUQUEUE_TIME_IDX] <= cur_time:
key, task = heapq.heappop(candidates)
ret.append(task[TASK_IDX])
cur_time += task[PROCESSING_TIME_IDX]
else:
cur_time = tasks[0][EUQUEUE_TIME_IDX]
# 잔여 태스트 모두 처리
while candidates:
key, task = heapq.heappop(candidates)
ret.append(task[TASK_IDX])
return ret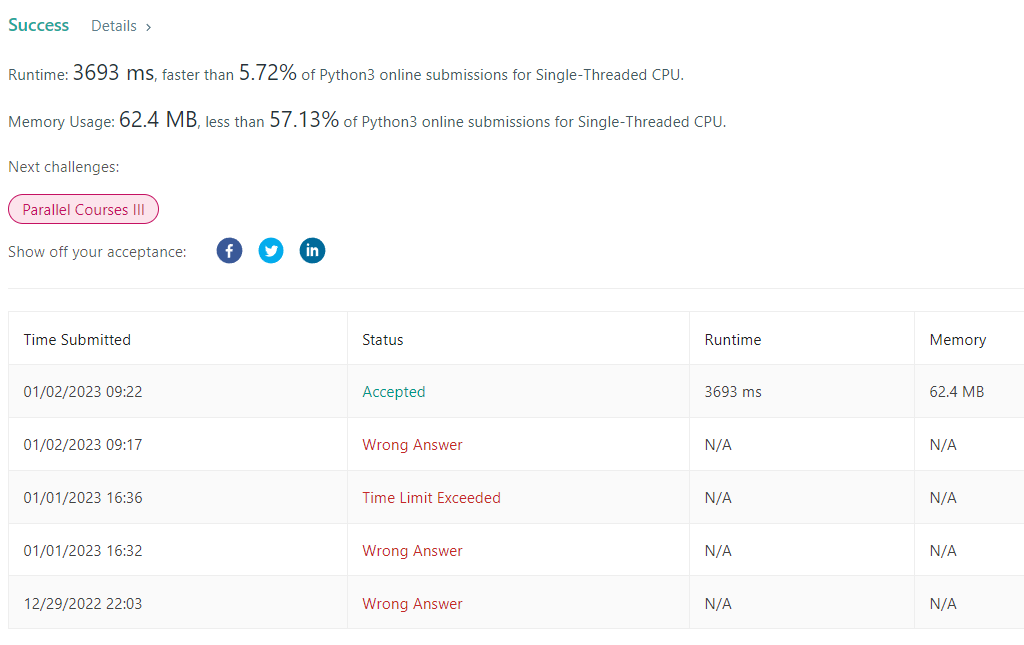
솔루션3)
- 솔루션3 리팩토링
class Solution:
def getOrder(self, tasks: List[List[int]]) -> List[int]:
EUQUEUE_TIME_IDX = 0
PROCESSING_TIME_IDX = 1
TASK_IDX = 2
# tasks with idx
tasks = [task + [idx] for idx, task in enumerate(tasks)]
# enqueueTime 오름차순 정렬
tasks = sorted(tasks, key=lambda x: x[EUQUEUE_TIME_IDX])
# 초기 시간 설정
cur_time = tasks[0][EUQUEUE_TIME_IDX]
ret = []
candidates = []
while candidates or len(tasks):
# 시간이 지나서 처리해야 할 태스크 후보 등록
while tasks and tasks[0][EUQUEUE_TIME_IDX] <= cur_time:
task = tasks[0]
heapq.heappush(candidates, [task[PROCESSING_TIME_IDX], task[TASK_IDX]])
del tasks[0]
# 태스트 처리
if candidates:
processing_time, task_idx = heapq.heappop(candidates)
ret.append(task_idx)
cur_time += processing_time
else:
cur_time = tasks[0][EUQUEUE_TIME_IDX]
return ret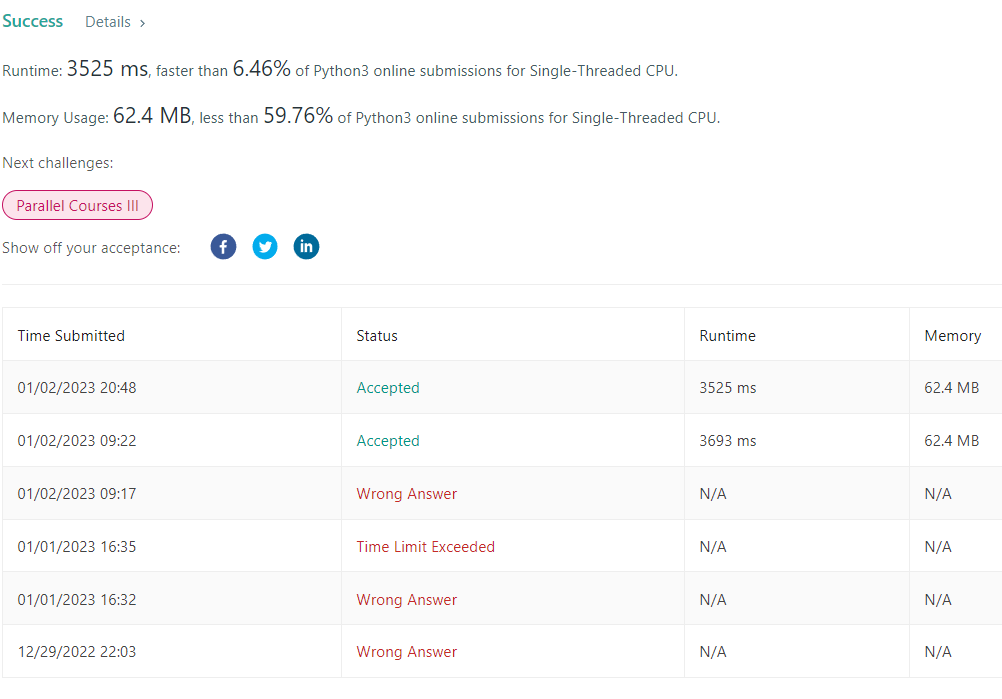
반응형
'알고리즘 > LeetCode' 카테고리의 다른 글
| 2233. Maximum Product After K Increments (0) | 2023.01.03 |
|---|---|
| 520. Detect Capital (0) | 2023.01.02 |
| 290. Word Pattern (0) | 2023.01.01 |
| 1534. Count Good Triplets (0) | 2022.12.29 |
| 1962. Remove Stones to Minimize the Total (0) | 2022.12.29 |
Comments